Custom Search
Natural Trigonometric Functions
Sine, Cosine and Tangent - Natural Trigonometric Functions
Sponsored Links
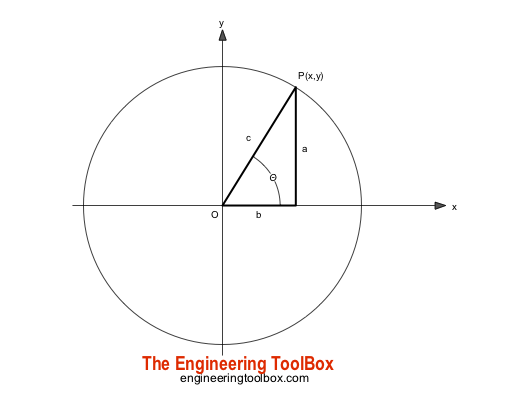
Natural trigonometric functions are expressed as
sin θ = cos (π / 2 - θ) (1)
cos θ = sin (π / 2 - θ) (2)
tan θ = sin θ / cos θ = 1 / cot θ = cot (π / 2 - θ) (3)
cot = 1 / tan θ = cos θ / sin θ = tan(π / 2 - θ) (4)
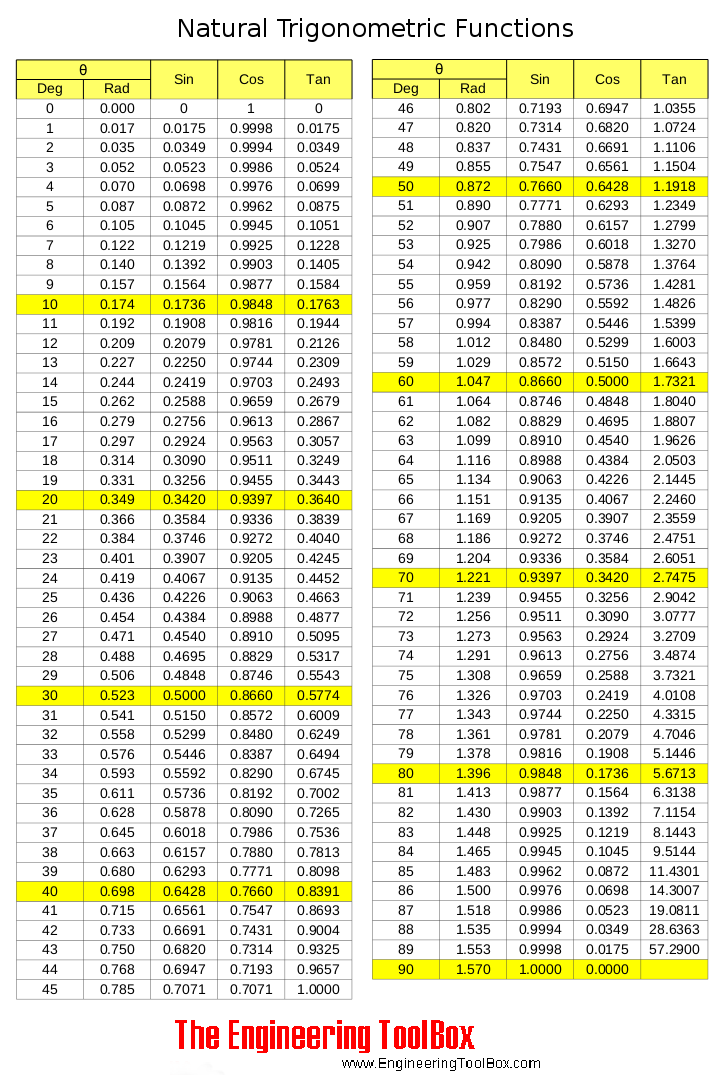
Trigonometric functions ranging 0 to 90 degrees are tabulated below:
Sponsored Links
Related Topics
- Basics - Basic Information as SI-system, Unit converters, Physical constants...
- Mathematics - Mathematical rules and laws - areas, volumes, exponents, trigometric functions and more
Sponsored Links
Related Documents
- Squares, Cubes and Roots - Numbers - squares, cubes, square roots and cube roots
