Engineering ToolBox - Resources, Tools and Basic Information for Engineering and Design of Technical Applications!

Hydrostatic Pressure vs. Depth

Depth and hydrostatic pressure.

hydrostatic pressure

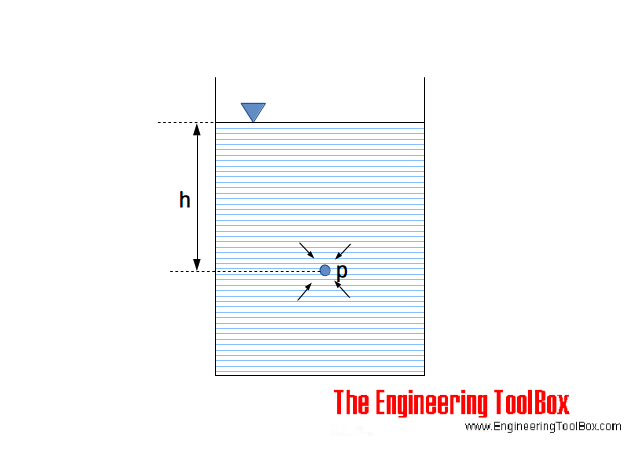
Hydrostatic pressure in a liquid can be calculated as

p = ρ g h                         (1)

where

p = pressure in liquid (N/m2, Pa, lbf /ft2, psf)

ρ = density of liquid (kg/m3, slugs /ft3 )

g = acceleration of gravity (9.81 m/s2, 32.17405 ft/s2)

h = height of fluid column - or depth in the fluid where pressure is measured (m, ft)

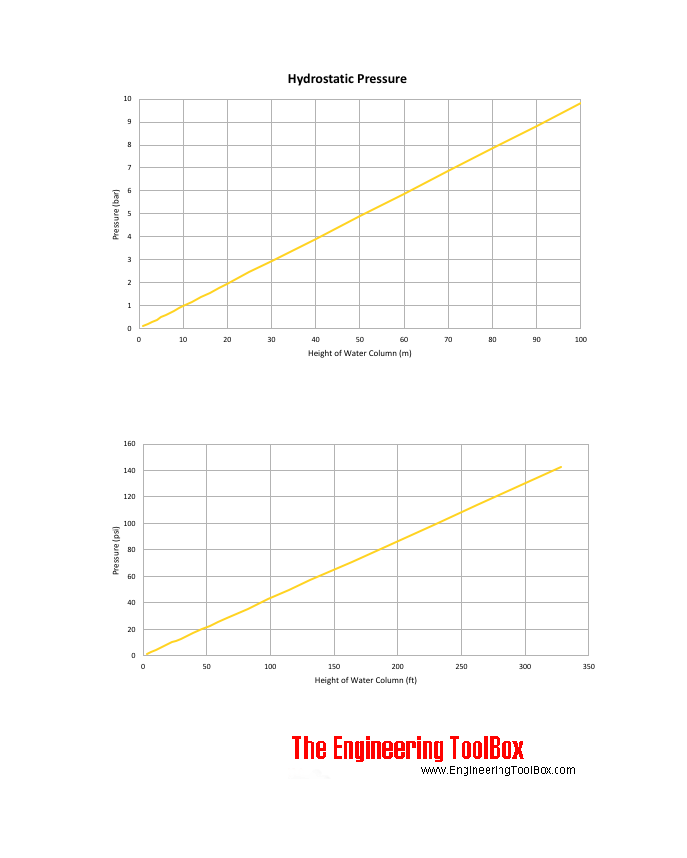
Hydrostatic pressure in a water column - or depth ( density of water 1000 kg/m3 ):

Hydrostatic Pressure vs. Water Depth
Height of Water ColumnPressure
(m)(ft)(kPa)(bar)(atm)(psi)
1 3.28 9.81 0.098 0.097 1.42
2 6.56 19.6 0.196 0.194 2.85
3 9.84 29.4 0.294 0.290 4.27
4 13.1 39.2 0.392 0.387 5.69
5 16.4 49.1 0.491 0.484 7.11
6 19.7 58.9 0.589 0.581 8.54
7 23.0 68.7 0.687 0.678 10.0
8 26.2 78.5 0.785 0.775 11.4
9 29.5 88.3 0.883 0.871 12.8
10 32.8 98.1 0.981 0.968 14.2
12 39.4 118 1.18 1.16 17.1
14 45.9 137 1.37 1.36 19.9
16 52.5 157 1.57 1.55 22.8
18 59.0 177 1.77 1.74 25.6
20 65.6 196 1.96 1.94 28.5
25 82.0 245 2.45 2.42 35.6
30 98.4 294 2.94 2.90 42.7
35 115 343 3.43 3.39 49.8
40 131 392 3.92 3.87 56.9
50 164 491 4.91 4.84 71.1
60 197 589 5.89 5.81 85.4
70 230 687 6.87 6.78 100
80 262 785 7.85 7.75 114
90 295 883 8.83 8.71 128
100 328 981 9.81 9.68 142

hydrostatic pressure water kPa Pa bar psi meter feet

Example - Pressure acting in water at depth 1 m

The density of water at 4 oC is 1000 kg/m3 . The pressure acting in water at 1 m can be calculated as

p = ρ g h

= ( 1000 kg/m3 ) ( 9.81 m/s2) (1 m)

= 9810 Pa

Example - Pressure acting in water at depth 3 ft

The density of water at 32 oF is 1.940 slugs/ft3 . The pressure acting in water at 3 ft can be calculated as

p = ρ g h

= ( 1.940 slugs/ft3 ) ( 32.17405 ft/s2) (3 ft)

= 187.3 lbf /ft2(psf)

= 1.3 lbf /in2(psi)

Ocean Pressure - Depth and Latitude

Ocean pressure varies with depth and position (latitude) on earth.

Ocean Pressure vs. Depth and Latitude

Depth
(m) 		Ocean Pressure (MPa)
Latitude (degrees)
0153045607590
0 0 0 0 0 0 0 0
1000 10.080 10.083 10.093 10.106 10.120 10.130 10.133
2000 20.208 20.215 20.234 20.261 20.288 20.308 20.315
3000 30.383 30.394 30.424 30.464 30.505 30.535 30.545
4000 40.606 40.620 40.660 40.714 40.768 40.808 40.823
5000 50.874 50.892 50.942 51.010 51.079 51.129 51.147
6000 61.188 61.210 61.270 61.352 61.434 61.495 61.517
7000 71.547 71.572 71.643 71.739 71.835 71.906 71.932
8000 81.949 81.979 82.059 82.170 82.280 82.361 82.391
9000 92.395 92.428 92.519 92.644 92.769 92.861 92.894
10000 102.880 102.917 103.019 103.157 103.296 103.398 103.436
  • 1 Pa = 10-6 MPa = 10-3 kPa = 10-6 N/mm2= 10 -5 bar = 0.1020 kp/m2= 1.020x10-4 m H2O at 4°C/39°F = 9.869x10-6 atm = 0.004 in H2O= 1.450x10-4 psi (lbf/in2) = 0.02089 lbf/ft2(psf)

Related Topics

  • Fluid Mechanics

    The study of fluids - liquids and gases. Involving velocity, pressure, density and temperature as functions of space and time.

Related Documents

Search

Search is the most efficient way to navigate the Engineering ToolBox.

Engineering ToolBox - SketchUp Extension - Online 3D modeling!

3D Engineering ToolBox Extension to SketchUp - add parametric components to your SketchUp model

Add standard and customized parametric components - like flange beams, lumbers, piping, stairs and more - to your Sketchup model with the Engineering ToolBox - SketchUp Extension - enabled for use with older versions of the amazing SketchUp Make and the newer "up to date" SketchUp Pro . Add the Engineering ToolBox extension to your SketchUp Make/Pro from the Extension Warehouse !

Translate this Page

Translate this page to Your Own Language .

About the Engineering ToolBox!

Privacy Policy

We don't collect information from our users. More about

We use a third-party to provide monetization technologies for our site. You can review their privacy and cookie policy here.

You can change your privacy settings by clicking the following button: .

Citation

This page can be cited as

  • The Engineering ToolBox (2010). Hydrostatic Pressure vs. Depth. [online] Available at: https://www.engineeringtoolbox.com/hydrostatic-pressure-water-d_1632.html [Accessed Day Month Year].

Modify the access date according your visit.

3D Engineering ToolBox - draw and model technical applications! 2D Engineering ToolBox - create and share online diagram drawing templates! Engineering ToolBox Apps - mobile online and offline engineering applications!

Unit Converter

















































9.26.9

.