Center Mass
Calculate position of center mass.
A coordinate system for determining center of mass:
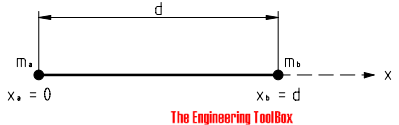
The position of center mass can calculated as
Rx = (ma xa + mb xb) / (ma + mb)
= (mb/ (ma + mb)) d (1)
where
Rx = x-coordinate for the center of mass
Engineering ToolBox - Resources, Tools and Basic Information for Engineering and Design of Technical Applications!
A coordinate system for determining center of mass:

The position of center mass can calculated as
Rx = (ma xa + mb xb) / (ma + mb)
= (mb/ (ma + mb)) d (1)
where
Rx = x-coordinate for the center of mass