Sound - Abatement vs. the Distance from Source
The disruption of the sound pressure wave and the reduction of noise is called attenuation - Sound Pressure Level vs. distance calculator.
The sound pressure from a source is reduced with distance from source.
Spherical Distance
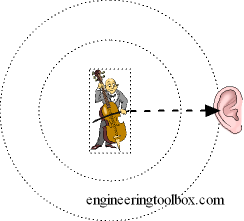
Sound pressure in spherical distance from a noise source can be calculated as:
p2 = ρ c N / (4 π r2) (1)
where
p = sound pressure (Pa, N/m2)
ρ = density of air (kg/m3)
c = speed of sound (m/s)
N = sound power (W)
π = 3.14
r = distance from source (m)
Half Spherical Distance
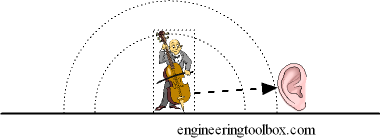
Sound pressure in half spherical distance from a source can be expressed as:
p2 = ρ c N / (4 π r2/ 2)
= 2 ρ c N / (4 π r2) (2)
A more generic expression for sound pressure in distance from source can be formulated to:
p2 = D ρ c N / (4 π r2) (3)
where
D = directivity coefficient (1 spherical, 2 half spherical)
The directivity coefficient depends on several parameters - the position and direction of the source, the room and the surrounding area, etc.
The Sound Pressure Level - Lp- can be expressed logarithmic in decibels as:
Lp = 20 log(p / pref)
= 20 log((D ρ c N / (4 π r2))1/2 / pref)
= 20 log(1 / r (D ρ c N / (4 π))1/2 / pref) (4)
where
Lp = sound pressure level (dB)
pref = 2×10-5 - reference sound pressure (Pa)
Note! - a doubling of the distance from a sound source - will reduce the sound pressure level - Lp - with 6 decibels.
Sound Pressure Level Calculator
Example - Sound Pressure from a Wood Planer
The sound power generated from a wood planer is estimated to 0.01 W. The sound pressure in distance 10 m from the planner can be calculated as
Lp = 20 log((D ρ c N / (4 π r2))1/2 / pref)
= 20 log(2 (1 kg/m3) (331.2 m/s) (0.01 W) / (4 π (10 m)2))1/2 / (2×10-5 Pa))
= 71 dB



